
ZhETF, Vol. 141,
No. 4,
p. 723 (April 2012)
(English translation - JETP,
Vol. 114, No. 4,
p. 631,
April 2012
available online at www.springer.com
)
DEVELOPMENT OF THE NEW APPROACH TO THE DIFFUSION-LIMITED REACTION RATE THEORY
Veshchunov M.S.
Received: July 20, 2011
The new approach to the diffusion-limited reaction rate theory, recently proposed by the author, is further developed on the base of a similar approach to Brownian coagulation. The traditional diffusion approach to calculation of the reaction rate is critically analyzed. In particular, it is shown that the traditional approach is applicable only in the special case of reactions with a large reaction radius, 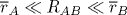 (where (where 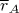 and and 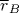 are the mean inter-particle distances), and becomes inappropriate in calculating the reaction rate in the case of a relatively small reaction radius, are the mean inter-particle distances), and becomes inappropriate in calculating the reaction rate in the case of a relatively small reaction radius, 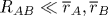 . In the latter case, most important for chemical reactions, particle collisions occur not in the diffusion regime but mainly in the kinetic regime characterized by homogeneous (random) spatial distribution of particles on the length scale of the mean inter-particle distance. The calculated reaction rate for a small reaction radius in three dimensions formally (and fortuitously) coincides with the expression derived in the traditional approach for reactions with a large reaction radius, but notably deviates at large times from the traditional result in the planar two-dimensional geometry. In application to reactions on discrete lattice sites, new relations for the reaction rate constants are derived for both three-dimensional and two-dimensional lattices. . In the latter case, most important for chemical reactions, particle collisions occur not in the diffusion regime but mainly in the kinetic regime characterized by homogeneous (random) spatial distribution of particles on the length scale of the mean inter-particle distance. The calculated reaction rate for a small reaction radius in three dimensions formally (and fortuitously) coincides with the expression derived in the traditional approach for reactions with a large reaction radius, but notably deviates at large times from the traditional result in the planar two-dimensional geometry. In application to reactions on discrete lattice sites, new relations for the reaction rate constants are derived for both three-dimensional and two-dimensional lattices.
|
|